Page 8 - Fen Lisesi Matematik 9 | 4. Ünite

ÜÇGENLER

9. ÖRNEK

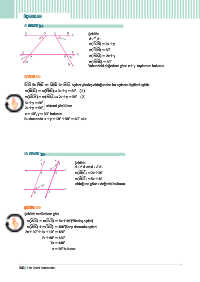
Şekilde
d1 ' d2
%
m RCN = x 3 + y
h
^
%
m PBN = 60c
h
^
%
m MAL = x 2 + y
h
^
%
m MDK = 50c
^
h
Yukarıdaki değerlere göre x + toplamını bulunuz.
y
ÇÖZÜM
% % % %
RCN ile PBN ve MDK ile MAL açıları yöndeş olduğundan bu açıların ölçüleri eşittir.
%
%
mRCN = mPBN & x 3 + = 60c ... 1 ] g
y
h
^
^
h
% %
y
mMDK = mMAL & x 2 + = 50c ... 2 ] g
h
^
h
^

y
x 3 += 60c 4 sistemi çözülürse
y
x 2 += 50c
x = 10c , y = 30c bulunur.
Bu durumda x += 10 + 30 = 40c olur.
y
c
c
10. ÖRNEK
Şekilde
d1 ' d ved3 ' d4
2
%
mABC = x 2 + 30c
^
h
%
mADE = x 5 + 10c
h
^
olduğuna göre x değerini bulunuz.

ÇÖZÜM
Şekilde verilenlere göre
% %
mADE = mBCE = x 5 + 10c (Yöndeş açılar)
^
h
^
h
% %
h
^
mABC + ^h mBCE = 180c(Karşı durumlu açılar)
c
c
x 2 + 30 + x 5 + 10 = 180c
x 7 + 40 = 180c
c
x 7 = 140c
x = 20c bulunur .
232 | Fen Lisesi Matematik 9