Page 160 - Matematik

12 Matematik

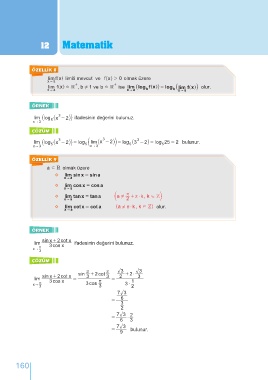
ÖZELLİK 8
0
limf x ] g limiti mevcut ve fx 2 olmak üzere
^ h
x " a
lim f x d R + , b ! 1 veb d R + ise lim logf] xgi = log _ lim ] olur .
_
] g
f xgi
x " a x " a b b x " a
ÖRNEK
3
2
lim log _ x - ij ifadesinin değerini bulunuz.
`
5
x " 3
ÇÖZÜM
3 3 3
2 =
lim ` log _ x - 2ij = log b lim _ x - 2il = log _ 3 - i log 25 = 2 bulunur .
5
5
5
5
x " 3 x " 3
ÖZELLİK 9
a d R olmak üzere
( x " a sina
limsinx =
( x " a cos a
limcosx =
( x " a tana b a ! r + $ r , k k d Zl
limtanx =
2
( lim cotx = cot a ^ a ! r $ , k k d Zh olur .

a
x "

ÖRNEK

lim sin x + 2 cot x ifadesinin değerini bulunuz.
cos x
3
r
x " 3
ÇÖZÜM

3
3
lim sin x + 2 cot x = sin r + 2 cot r = 2 3 + 2 $ 3 3
1
3
cos x
r
r 3 cos 3 $
x " 3 3 2
73
6
=
3
2
73 2
= 6 $ 3
73
= 9 bulunur .

160